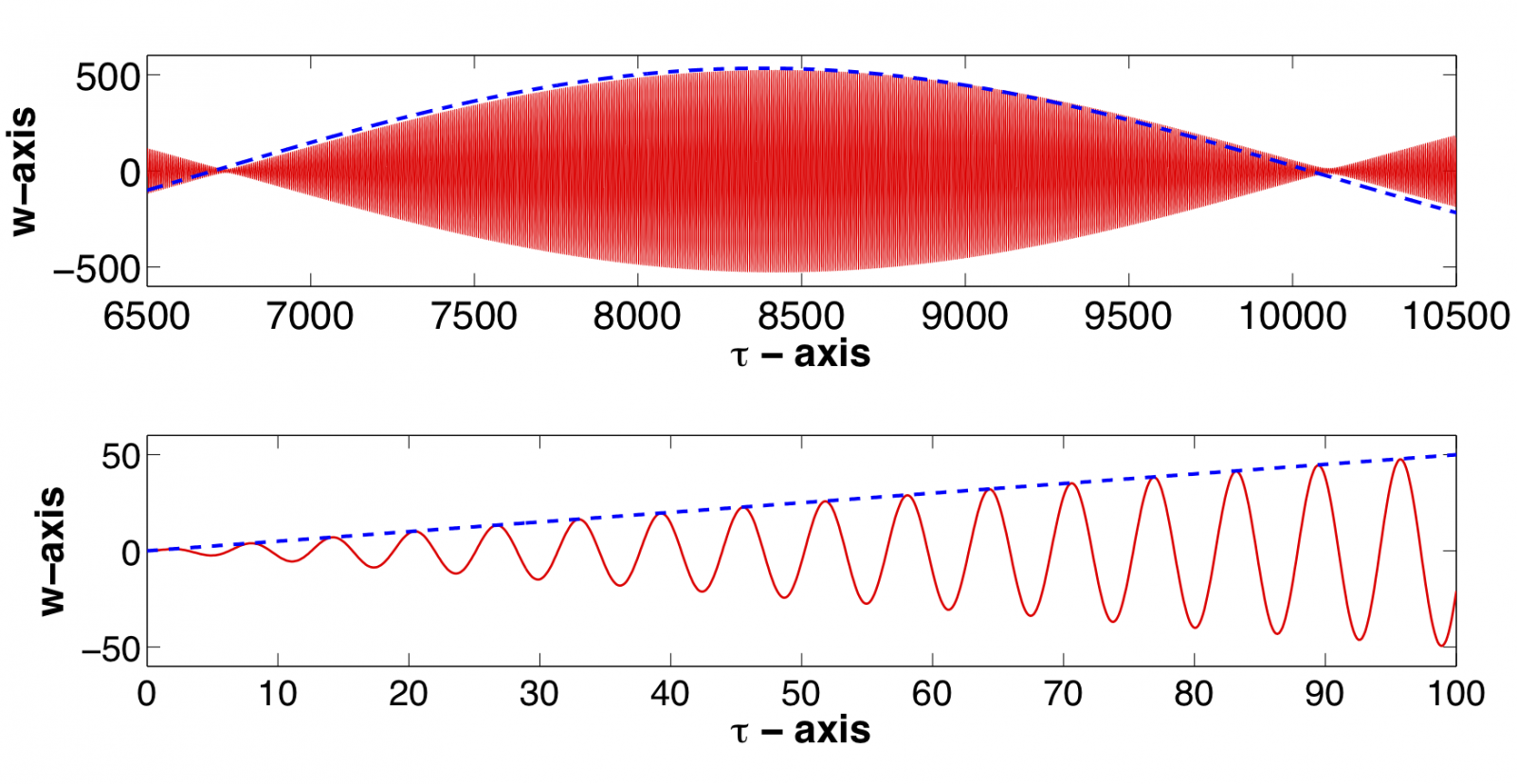
Introduction
The fundamental open question in understanding how we hear concerns the role of a nonlinear feedback mechanism known as the cochlear amplifier. We are developing approximation methods that can be used to help study this problem, both the mechanistic basis for amplification as well as its effects on wave propagation in the cochlea. The underlying assumption is that there are two principal geometric regimes within the cochlea, and they are strongly coupled. One of the regimes gives rise to a macroscale problem. Our hydroelastic model effectively constitutes a first-order approximation to the solution in this regime, except that the equation for the partition that contains the cells responsible for transduction must be modified to account for amplification. The other regime is associated with the microscale, and concerns the motion within the partition. Homogenization is used to derive an effective equation for the partition from the microstructure, and this is used in the macroscale problem.
Affiliated Researchers
Past Graduate Research Assistants:
- Kimberly Fessel
Resources
Selected Publications:
- Conservative numerical methods for nonlinear oscillators, M. H. Holmes, American Journal of Physics, to appear (2019).
- A model and analysis for the nonlinear amplification of waves in the cochlea, K. Fessel and M. H. Holmes, Mathematical Biosciences, v301, July 2018, 10-20.
- Connections Between Cubic Splines and Quadrature Rules, M. H. Holmes, American Mathematical Monthly, v121(8), 2014, 723-726.
- A model for the nonlinear mechanism responsible for cochlear amplification, K. Fessel and M. H. Holmes, Mathematical Biosciences and Engineering, v11(6), 2014, 1357-1373.
- Asymmetric random walks and drift-diffusion, M. H. Holmes, Europhysics Letters (EPS), v102(3), 2013.