Introduction
This is an interdisciplinary program for undergraduates, graduate students, and postdoctoral associates that integrates modeling, analysis, and computations with contemporary experimental research. It is centered on large multi-scale nonlinear systems and the mathematical and numerical methods needed to analyze them. Essential components of this effort are the introduction of emerging research methods into the curriculum and a much closer interaction with researchers outside mathematics. This enriches the educational and professional experiences of our students and postdocs, with the result that they are strong researchers who are able to work on emerging real-world problems in science and engineering.
Affiliated Researchers
Current Postdoctoral Research Fellows:
- Joe Klobusicky
- Derek Olson
Current Graduate Research Assistants:
- Paulina Volosov
- Michael Hennessey
- Arthur Newell
Past Postdoctoral Research Fellows:
- Michael Jenkinson
- David Wells
Past Graduate Research Assistants:
- Jordan Angel
- Karen Cumings
- Jennifer Kile-Crodelle
- Katelyn Plaisier-Leisman
- Yosef Treitman
- Michael Caiola
- Michael Schwarz
- John Jacangelo
Resources
Selected Publications:
- A model and analysis for the nonlinear amplification of waves in the cochlea K. Fessel and M. H. Holmes, Mathematical Biosciences, v301, July 2018, 10-20.
- Invariance properties for the error function used for multilinear regression M. Caiola and M. H. Holmes, PLOS ONE, Dec 2018
- Invariance properties for the error function used for multilinear regression M. Caiola and M. H. Holmes, PLOS ONE, Dec 2018
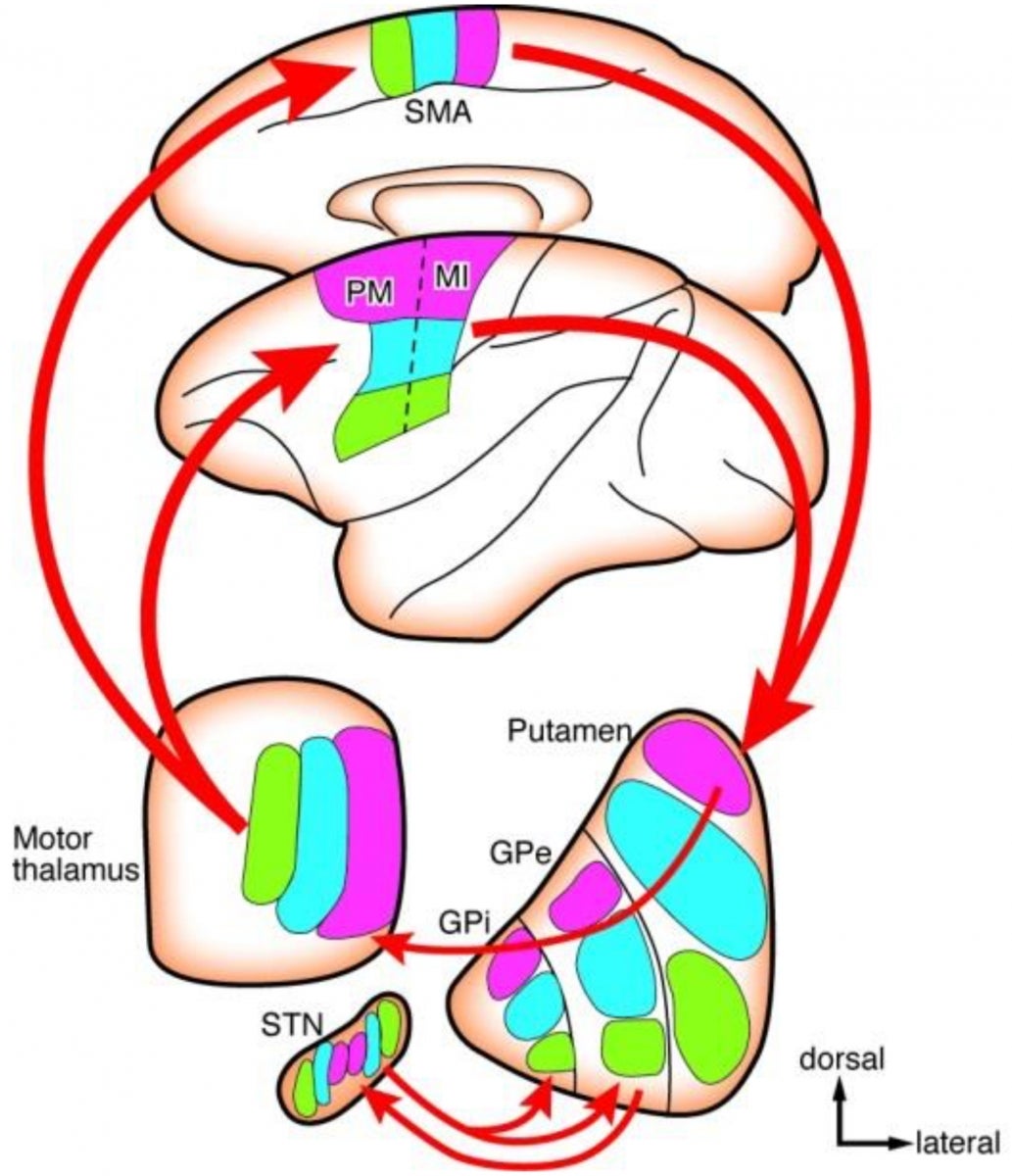
- Model and Analysis for the Onset of Parkinsonian Firing Patterns in a Simplified Basal Ganglia M. Caiola and M. H. Holmes, International Journal of Neural Systems, 2019, v29(01)